Author:
(1) Yuki Koto
Table of Links
- Abstract and Intro
- Genus-zero Gromov-Witten Theory
- Toric Bundles
- Lagrangian cones of Toric bundles
- Mirror theorem for a product of projectives bundles
- Mirror Theorem for Toric Bundles
- Appendix A. Equivariant Fourier Transformation and References
Abstract
We construct an I-function for toric bundles obtained as a fiberwise GIT quotient of a (not necessarily split) vector bundle. This is a generalization of Brown’s I-function for split toric bundles [5] and the I-function for non-split projective bundles [21]. In order to prove the mirror theorem, we establish a characterization of points on the Givental Lagrangian cones of toric bundles and prove a mirror theorem for the twisted Gromov-Witten theory of a fiber product of projective bundles. The former result generalizes Brown’s characterization for split toric bundles [5] to the non-split case.
1. Introduction
The genus-zero Gromov-Witten theory of a smooth projective variety X plays a significant role in symplectic geometry, algebraic geometry and mirror symmetry. It can be studied by a mirror theorem [13], that is, by finding a convenient point (called an I-function) on the Givental Lagrangian cone LX [14]. The cone LX is a Lagrangian submanifold of an infinite-dimensional symplectic vector space HX, called the Givental space, and is defined by genus-zero gravitational Gromov-Witten invariants. A mirror theorem for X enables us to compute genus-zero Gromov-Witten invariants of X and study quantum cohomology.
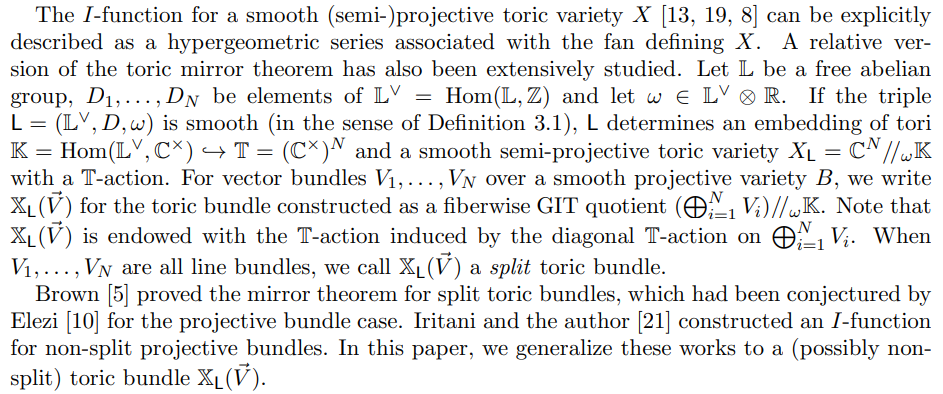
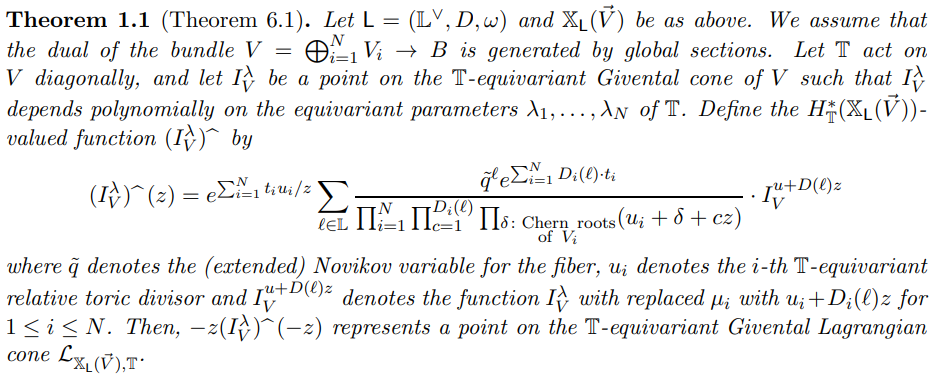
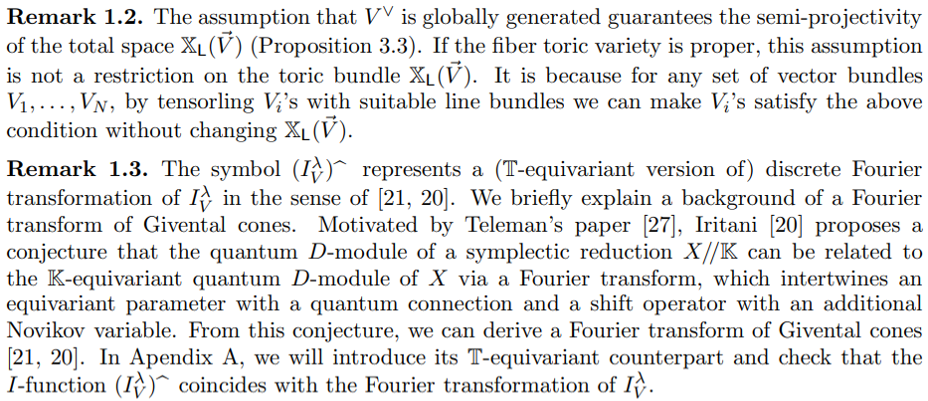
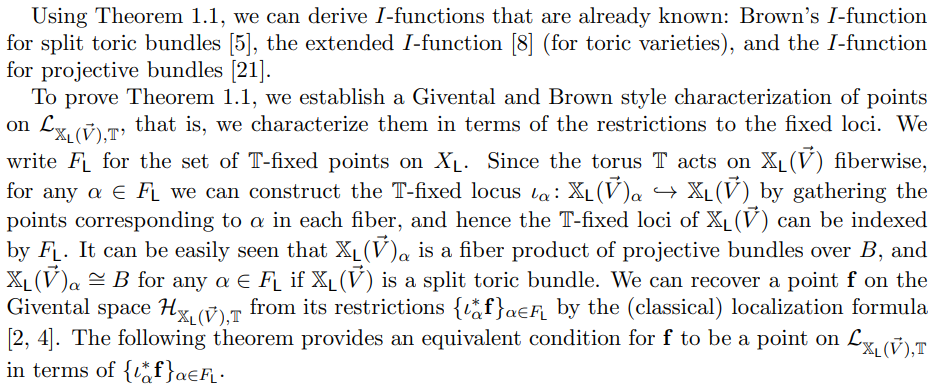

This is a generalization of Brown’s result [5, Theorem 2], which gives the same characterization for split toric bundles. There are also similar characterization results for other varieties/stacks; see [8, 23, 11].
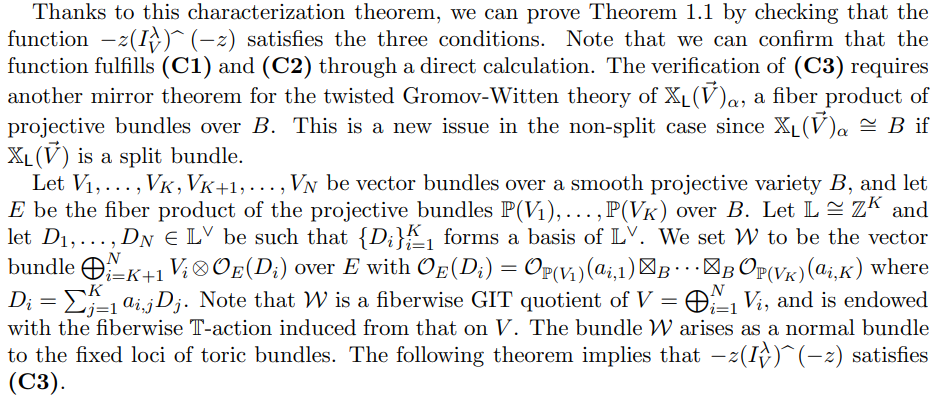
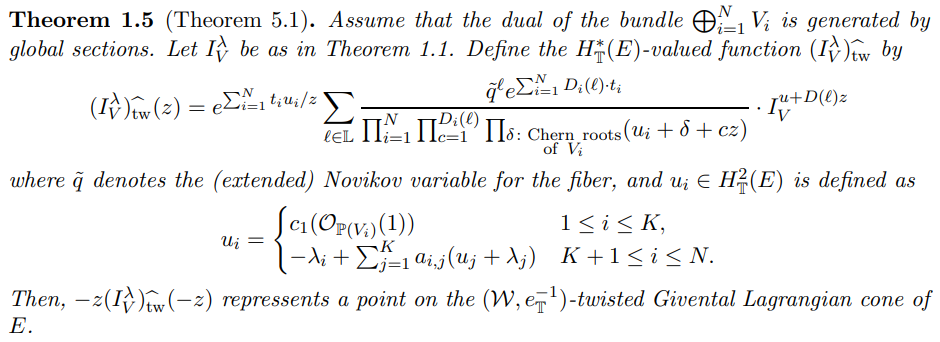

This result is a straightforward generalization of the mirror theorem for non-split projective bundles [21, Theorem 3.3]. The key ingredient of the proof is the quantum Riemann-Roch theorem [9, Corollary 4] and the well-known fact [24] that Gromov-Witten invariants of the zero locus of a regular section of a convex vector bundle over a variety X are given by twisted Gromov-Witten invariants of X.
The plan of the paper is as follows. In Section 2, we recall the definition of GromovWitten invariants, and introduce the non-equivariant/equivariant/twisted Givental cones and quantum Riemann-Roch theorem. In Section 3, we introduce the notion of split/non-split toric bundles, and summarize the structure of cohomology and the semigroups generated by effective curve classes, which will be needed in the subsequent sections. In Section 4, we establish a characterization theorem (Theorem 4.2) for points on the Lagrangian cone of a toric bundle. In Section 5, we prove a mirror theorem for twisted Gromov-Witten theory of a fiber product of projective bundles over B. In Section 6, we prove the main result (Theorem 6.1) of this paper, that is, a mirror theorem for (possibly non-split) toric bundles. In Appendix A, we briefly explain a Fourier transform of Givental cones, and check that our I-function coincides with the Fourier transform of the I-function of a vector bundle.
Acknowledgements. The author is deeply grateful to Hiroshi Iritani for his guidance and enthusiastic support during the writing of this paper. He also would like to thank Yuan-Pin Lee and Fumihiko Sanda for very helpful discussions. This work was supported by JSPS KAKENHI Grant Number 22KJ1717.
This paper is available on arxiv under CC 4.0 license.

