Author:
(1) Yuki Koto
Table of Links
- Abstract and Intro
- Genus-zero Gromov-Witten Theory
- Toric Bundles
- Lagrangian cones of Toric bundles
- Mirror theorem for a product of projectives bundles
- Mirror Theorem for Toric Bundles
- Appendix A. Equivariant Fourier Transformation and References
Appendix A. Equivariant Fourier transformation
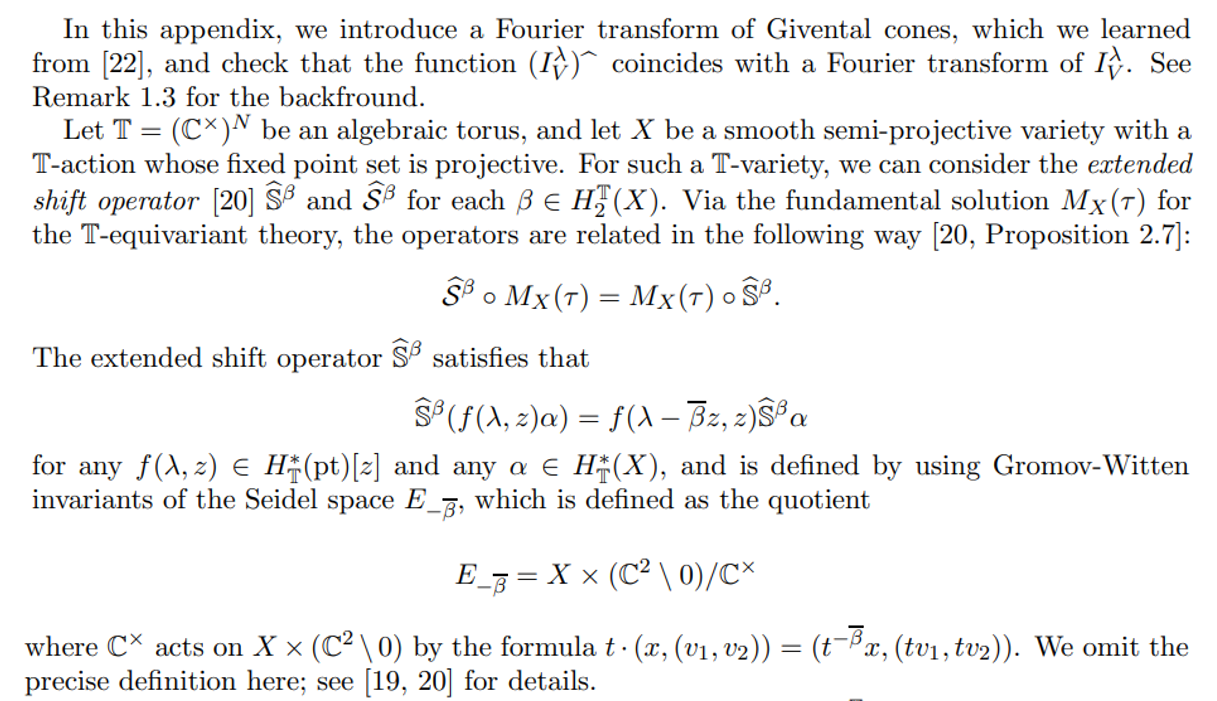
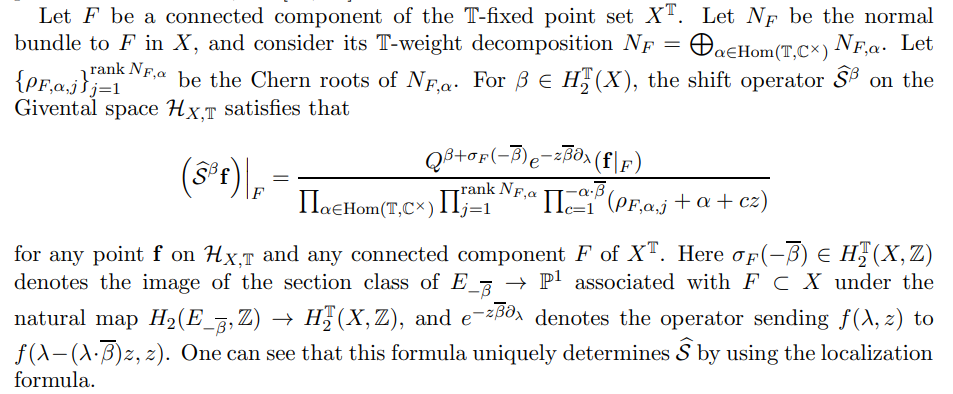
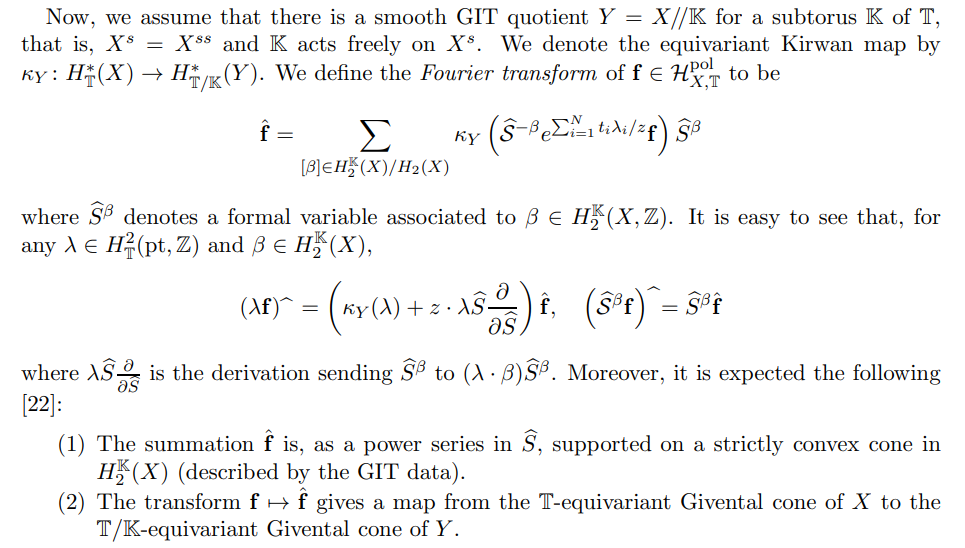
Note that this is a straightforward generalization of [20, Conjecture 1.7].
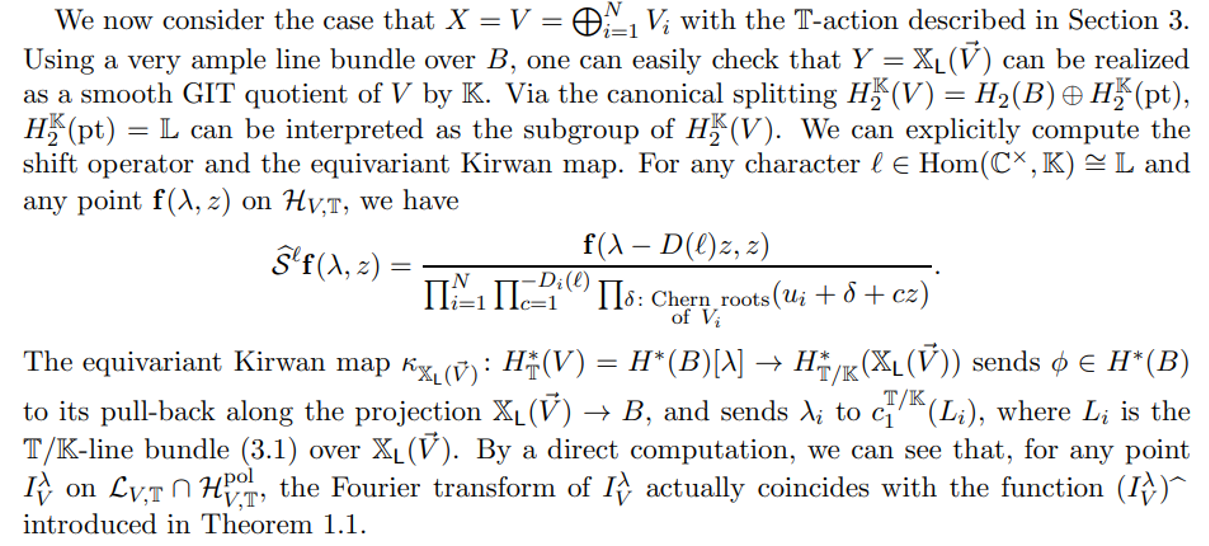
References
-
Dan Abramovich, Tom Graber, and Angelo Vistoli, Gromov-Witten theory of Deligne-Mumford stacks, Amer. J. Math. 130 (2008), no. 5, 1337–1398.
-
M. F. Atiyah and R. Bott, The moment map and equivariant cohomology, Topology 23 (1984), no. 1, 1–28.
-
K. Behrend, Gromov-Witten invariants in algebraic geometry, Invent. Math. 127 (1997), no. 3, 601–617.
-
Nicole Berline and Mich`ele Vergne, Classes caract´eristiques ´equivariantes. Formule de localisation en cohomologie ´equivariante, C. R. Acad. Sci. Paris S´er. I Math. 295 (1982), no. 9, 539–541.
-
Jeff Brown, Gromov-Witten invariants of toric fibrations, Int. Math. Res. Not. IMRN (2014), no. 19, 5437–5482.
-
Charles Cadman, Using stacks to impose tangency conditions on curves, Amer. J. Math. 129 (2007), no. 2, 405–427.
-
Tom Coates, Alessio Corti, Hiroshi Iritani, and Hsian-Hua Tseng, Computing genus-zero twisted GromovWitten invariants, Duke Math. J. 147 (2009), no. 3, 377–438.
-
_________, A mirror theorem for toric stacks, Compos. Math. 151 (2015), no. 10, 1878–1912.
-
Tom Coates and Alexander Givental, Quantum Riemann-Roch, Lefschetz and Serre, Ann. of Math. (2) 165 (2007), no. 1, 15–53.
-
Artur Elezi, A mirror conjecture for projective bundles, Int. Math. Res. Not. (2005), no. 55, 3445–3458.
-
Honglu Fan and Yuan-Pin Lee, On Gromov-Witten theory of projective bundles, Michigan Math. J. 69 (2020), no. 1, 153–178.
-
William Fulton, Intersection theory, second ed., Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], vol. 2, Springer-Verlag, Berlin, 1998.
-
Alexander Givental, A mirror theorem for toric complete intersections, Topological field theory, primitive forms and related topics (Kyoto, 1996), Progr. Math., vol. 160, Birkh¨auser Boston, Boston, MA, 1998, pp. 141–175.
-
Alexander B. Givental, Symplectic geometry of Frobenius structures, Frobenius manifolds, Aspects Math., vol. E36, Friedr. Vieweg, Wiesbaden, 2004, pp. 91–112.
-
T. Graber and R. Pandharipande, Localization of virtual classes, Invent. Math. 135 (1999), no. 2, 487–518.
-
Tam´as Hausel and Bernd Sturmfels, Toric hyperK¨ahler varieties, Doc. Math. 7 (2002), 495–534.
-
Hiroshi Iritani, An integral structure in quantum cohomology and mirror symmetry for toric orbifolds, Adv. Math. 222 (2009), no. 3, 1016–1079.
-
_________, Quantum cohomology and periods, Ann. Inst. Fourier (Grenoble) 61 (2011), no. 7, 2909–2958.
-
_________, Shift operators and toric mirror theorem, Geom. Topol. 21 (2017), no. 1, 315–343.
-
Hiroshi Iritani, Quantum cohomology of blowups, 2023.
-
Hiroshi Iritani and Yuki Koto, Quantum cohomology of projective bundles, 2023, arXiv:2307.03696 [math.AG].
-
Hiroshi Iritani and Fumihiko Sanda, private communication.
-
Yunfeng Jiang, Hsian-Hua Tseng, and Fenglong You, The quantum orbifold cohomology of toric stack bundles, Lett. Math. Phys. 107 (2017), no. 3, 439–465.
-
Bumsig Kim, Andrew Kresch, and Tony Pantev, Functoriality in intersection theory and a conjecture of Cox, Katz, and Lee, J. Pure Appl. Algebra 179 (2003), no. 1-2, 127–136.
-
Chiu-Chu Melissa Liu, Localization in Gromov-Witten theory and orbifold Gromov-Witten theory, Handbook of moduli. Vol. II, Adv. Lect. Math. (ALM), vol. 25, Int. Press, Somerville, MA, 2013, pp. 353–425.
-
Rahul Pandharipande, Rational curves on hypersurfaces (after A. Givental), no. 252, 1998, S´eminaire Bourbaki. Vol. 1997/98, pp. Exp. No. 848, 5, 307–340.
-
Constantin Teleman, Gauge theory and mirror symmetry, Proceedings of the International Congress of Mathematicians—Seoul 2014. Vol. II, Kyung Moon Sa, Seoul, 2014, pp. 1309–1332.
-
Valentin Tonita, Twisted orbifold Gromov-Witten invariants, Nagoya Math. J. 213 (2014), 141–187.
-
Angelo Vistoli, Intersection theory on algebraic stacks and on their moduli spaces, Invent. Math. 97 (1989), no. 3, 613–670.
This paper is available on arxiv under CC 4.0 license.

